
The mean score is 60 out of 100 and the standard deviation (in other words, the variation in the scores) is 15 marks (see our statistical guides, Measures of Central Tendency and Standard Deviation, for more information about the mean and standard deviation). We make the assumption that when the scores are presented on a histogram, the data is found to be normally distributed. Setting the scene: Part 1Ī tutor sets a piece of English Literature coursework for the 50 students in his class. To explain what this means in simple terms, let's use an example (if needed, see our statistical guide, Normal Distribution Calculations, for background information on normal distribution calculations).
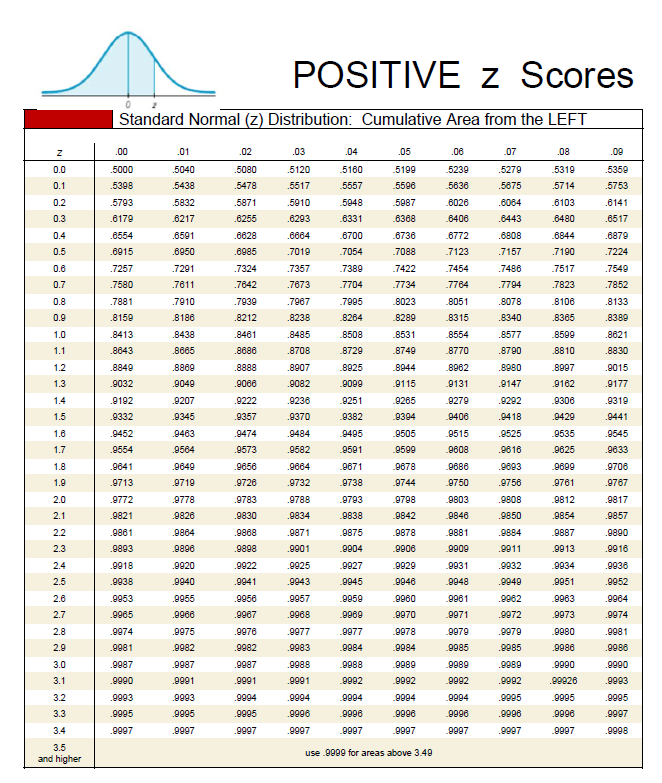
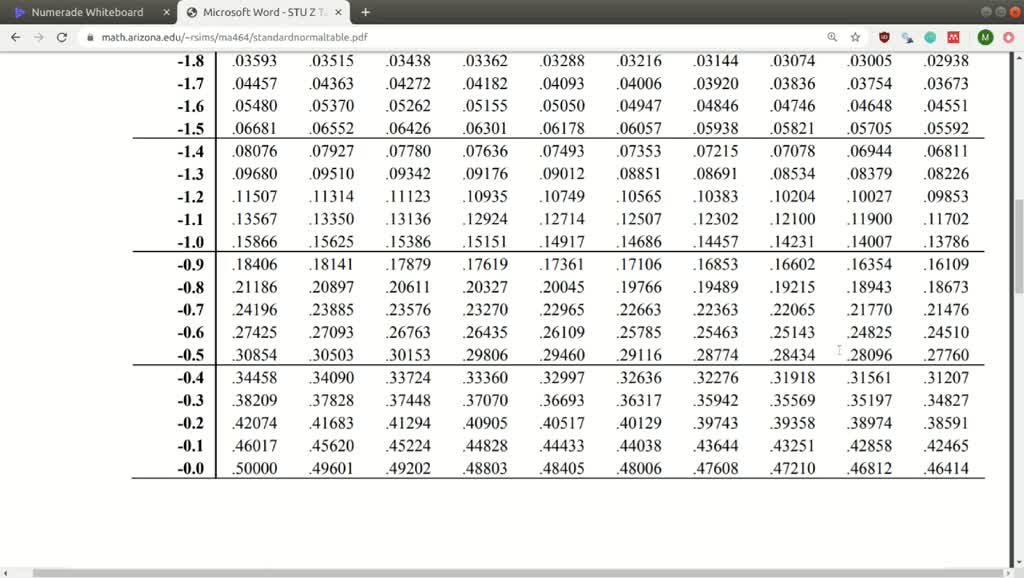
The standard score does this by converting (in other words, standardizing) scores in a normal distribution to z-scores in what becomes a standard normal distribution. The standard score (more commonly referred to as a z-score) is a very useful statistic because it (a) allows us to calculate the probability of a score occurring within our normal distribution and (b) enables us to compare two scores that are from different normal distributions.


 0 kommentar(er)
0 kommentar(er)
